long division reasoning and problem solving

• Home   |  
• About   |  
• Contact Us   |  
• Privacy   |  
• Newsletter   |  
• Shop   |  
• 🔍 Search Site
• Christmas Worksheets
• Kindergarten
• All Generated Sheets
• Place Value Generated Sheets
• Addition Generated Sheets
• Subtraction Generated Sheets
• Multiplication Generated Sheets
• Division Generated Sheets
• Money Generated Sheets
• Negative Numbers Generated Sheets
• Fraction Generated Sheets
• Place Value Zones
• Number Bonds
• Addition & Subtraction
• Times Tables
• Fraction & Percent Zones
• All Calculators
• Fraction Calculators
• Percent calculators
• Area & Volume Calculators
• Age Calculator
• Height Calculator
• Roman Numeral Calculator
• Coloring Pages
• Fun Math Sheets
• Math Puzzles
• Mental Math Sheets
• Online Times Tables
• Online Addition & Subtraction
• Math Grab Packs
• All Math Quizzes
• Kindergarten Math Quizzes
• 1st Grade Quizzes
• 2nd Grade Quizzes
• 3rd Grade Quizzes
• 4th Grade Quizzes
• 5th Grade Quizzes
• 6th Grade Math Quizzes
• Place Value
• Rounding Numbers
• Comparing Numbers
• Number Lines
• Prime Numbers
• Negative Numbers
• Roman Numerals
• Subtraction
• Add & Subtract
• Multiplication
• Fraction Worksheets
• Learning Fractions
• Fraction Printables
• Percent Worksheets & Help
• All Geometry
• 2d Shapes Worksheets
• 3d Shapes Worksheets
• Shape Properties
• Geometry Cheat Sheets
• Printable Shapes
• Coordinates
• Measurement
• Math Conversion
• Statistics Worksheets
• Bar Graph Worksheets
• Venn Diagrams
• All Word Problems
• Finding all possibilities
• Logic Problems
• Ratio Word Problems
• All UK Maths Sheets
• Year 1 Maths Worksheets
• Year 2 Maths Worksheets
• Year 3 Maths Worksheets
• Year 4 Maths Worksheets
• Year 5 Maths Worksheets
• Year 6 Maths Worksheets
• All AU Maths Sheets
• Kindergarten Maths Australia
• Year 1 Maths Australia
• Year 2 Maths Australia
• Year 3 Maths Australia
• Year 4 Maths Australia
• Year 5 Maths Australia
• Meet the Sallies
• Certificates

Long Division Problems with Answers

(randomly generated).

Welcome to our Long Division Problems with Answers page.

Here you will find our free worksheet generator for generating your own long division sheets and answers.

If you are looking to create your own custom-made long or short division worksheets then look no further!

We also have several worked examples showing long division on this page so you can see how the method works.

For full functionality of this site it is necessary to enable JavaScript.

Here are the instructions how to enable JavaScript in your web browser .

Using the random sheet generator will allow you to:

• choose the number range and number of questions you wish the worksheet to have;
• print or save your worksheet and a corresponding answer sheet;
• choose your own title and instructions for completing the sheet - great for homework!

For optimal printing, please set your margins to zero on your print setup options.

To save your worksheets, select Print to PDF in the printing options.

If you have any problems with our Random Generator, please let us know using the Contact Us link at the top of each page.

Mobile View of Worksheets

Please note that our generated worksheets may have problems displaying correctly on some mobile devices.

This should not affect the printing of the sheets which should display correctly.

Long Division Problems with Answers Generator

Here is our random worksheet generator for creating your own long or short division worksheets.

You can generate a range of long division worksheets ranging from 2 digit by 1 digit up to 5 digits by 2 digits.

Using this generator will let you create your own worksheets for:

• Dividing 2, 3, 4 and 5 digit numbers by a range of different numbers up to 99;
• Dividing numbers with or without a remainder;
• Choosing exactly which divisors you want to include;

One of the best features of this generator is that you can choose individual values for the divisors for the worksheets (between 2 and 15).

This means that:

• if you were introducing long division for the first time, you could choose just to divide 2 digit numbers by, say, 2, 3 and 5.
• if you wanted to introduce dividing by two digits but you wanted to divide 3 digit numbers by 11 and 12, you could do that also.
• to access this feature, go to the 'Select divisors from a list' option.

To start creating your sheet, choose an option from the Number values box below.

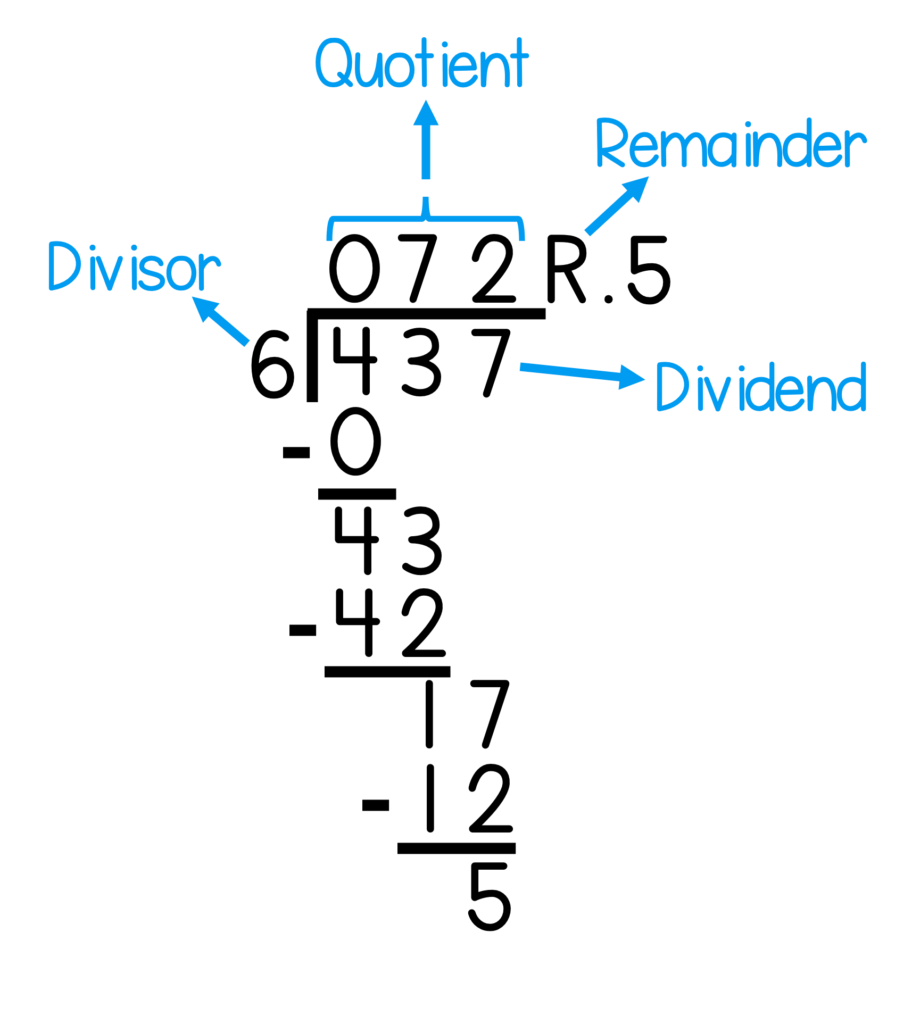
Parts of a Long Division Equation

The dividend is the number being divided.

The divisor is the number you are dividing by.

The quotient is the number of times the divisor goes into the dividend.

The remainder is what is left over from the dividend when the divisor has been taken away as many times as it can be without leading to a negative answer.

The remainder can be a whole number, but it can also be expressed as a fraction or decimal.

Long Division Problems with Answers Worksheet Generator

4 Steps to Your Worksheets...

• Choose your divisor value - this is the number you are dividing by
• Choose your dividend value - this is the number you are dividing
• Choose if you want to have remainders or not
• Choose the number of questions
• View your sheet
• Print your sheet (if you want to save a hard copy of your sheet, choose 'Save to PDF'.

(Optional) Give your worksheet a title.

(Optional) Write out any instructions to go at the top of the sheet.

Divisor Value:

Remainders:

Dividend value:

Number of Questions:

Your worksheet will appear below.

Examples of Long Division Problems with Answers

Here are some examples showing some long division problems with answers, along with commentary on each step.

Example 1) Work out 84 ÷ 3

So we are working out \[ 3 \enclose {longdiv} {84} \]

FIrst step is to work out 8 ÷ 3, which means we need to find how many groups of 3 make 8.

We can get 2 groups of 3 in 8, and 2 x 3 = 6 so this gives us: \[ \begin {array}{l} \quad 2 \\ 3 \enclose {longdiv} {84} \\ \quad \underline{6} \end{array} \]

Next we subtract 6 from 8 to give us: \[ \begin {array}{l} \quad 2 \\ 3 \enclose {longdiv} {84} \\ \quad \underline{6} \\ \quad 2 \end{array} \]

The next step is to bring down the 4 and put it next to the 2 \[ \begin {array}{l} \quad 2 \\ 3 \enclose {longdiv} {84} \\ \quad \underline{6} \\ \quad 24 \end{array} \]

We now have to work out 24 ÷ 3, or how many groups of 3 make 24.

8 groups of 3 make 24. So this gives us: \[ \begin {array}{l} \quad 28 \\ 3 \enclose {longdiv} {84} \\ \quad \underline{6} \\ \quad 24 \\ \quad \underline{24} \end{array} \]

Finally subtract 24 from 24 to give us an answer of 0. \[ \begin {array}{l} \quad 28 \\ 3 \enclose {longdiv} {84} \\ \quad \underline{6} \\ \quad 24 \\ \quad \underline{24} \\ \quad \; \; 0 \end{array} \]

There are no more digits to bring down and we have no remainders, so we have finished.

Answer: 84 ÷ 3 = 28

Example 2) Work out 347 ÷ 4

So we are working out \[ 4 \enclose {longdiv} {347} \]

FIrst step is to work out 3 ÷ 4, which means we need to find how many groups of 4 make 3.

There are 0 groups of 4 in 3, so we keep the 3 in its place and go on to the next digit which is 4.

This gives us: \[ \begin {array}{l} \quad 0 \\ 4 \enclose {longdiv} {347} \end{array} \]

So the next step is to work out 34 ÷ 4, or find how many groups of 4 make 34.

8 groups of 4 make 32. So this gives us: \[ \begin {array}{l} \quad 08 \\ 4 \enclose {longdiv} {347} \\ \quad \underline {32} \end{array} \]

We subtract 32 from 34 to give us an answer of 2. This gives us: \[ \begin {array}{l} \quad 08 \\ 4 \enclose {longdiv} {347} \\ \quad \underline {32} \\ \quad \; \; 2 \end{array} \]

Next we bring the 7 down alongside the 2. \[ \begin {array}{l} \quad 08 \\ 4 \enclose {longdiv} {347} \\ \quad \underline {32} \\ \quad \; \; 27 \end{array} \]

So we need to work out 27 ÷ 4, or find how many 4s make 27.

6 groups of 4 make 24. So this gives us: \[ \begin {array}{l} \quad 086 \\ 4 \enclose {longdiv} {347} \\ \quad \underline {32} \\ \quad \; \; 27 \\ \quad \; \; \underline {24} \end{array} \]

Finally we need to subtract 24 from 27 to give us 3.

There are no more digits to bring down, so we are left with 3 as a remainder. \[ \begin {array}{l} \quad 086r3 \\ 4 \enclose {longdiv} {347} \\ \quad \underline {32} \\ \quad \; \; 27 \\ \quad \; \; \underline {24} \\ \quad \quad 3 \end{array} \]

Answer: 347 ÷ 4 = 86r3

If we wanted the remainder as a fraction, we would have 347 ÷ 4 = 86 ¾

Example 3) Work out 8165 ÷ 12

So we are working out \[ 12 \enclose {longdiv} {8165} \]

FIrst step is to work out 8 ÷ 12, which means we need to find how many groups of 12 make 8.

There are 0 groups of 12 in 8, so we keep the 8 in its place and go on to the next digit which is 1.

This gives us: \[ \begin {array}{l} \quad \; 0 \\ 12 \enclose {longdiv} {8165} \end{array} \]

So the next step is to work out 81 ÷ 12, or find how many groups of 12 make 81.

6 groups of 12 make 72. So this gives us: \[ \begin {array}{l} \quad \; 06 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \end{array} \]

6 groups of 12 make 72. So this gives us: \[ \begin {array}{l} \quad \; 06 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 9 \end{array} \]

Next we bring the 6 down alongside the 9. \[ \begin {array}{l} \quad \; 06 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 96 \end{array} \]

So we need to work out 96 ÷ 12, or find how many 12s make 96.

8 groups of 12 make 96. So this gives us: \[ \begin {array}{l} \quad \; 068 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 96 \\ \qquad \underline{96} \end{array} \]

Now we subtract 96 from 96 to give us 0. \[ \begin {array}{l} \quad \; 068 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 96 \\ \qquad \underline{96} \\ \qquad \; \: 0 \end{array} \]

Now we bring down the 5. \[ \begin {array}{l} \quad \; 068 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 96 \\ \qquad \underline{96} \\ \qquad \; \: 05 \end{array} \]

Nexy we need to work out 5 ÷ 12, or how many 12s make 5.

0 groups of 12 make 5, so this gives us: \[ \begin {array}{l} \quad \; 0680 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 96 \\ \qquad \underline{96} \\ \qquad \; \: 05 \\ \qquad \; \: \underline {00} \end{array} \]

Subtracting 0 from 5 gives us 5. There are no more digits to bring down, so 5 is the remainder. \[ \begin {array}{l} \quad \; 0680r5 \\ 12 \enclose {longdiv} {8165} \\ \quad \; \, \underline {72} \\ \qquad 96 \\ \qquad \underline{96} \\ \qquad \; \: 05 \\ \qquad \; \: \underline {00} \\ \qquad \quad 5 \end{array} \]

Answer: 8165 ÷ 12 = 680r5

If we wanted the remainder as a fraction, we would have \[ 8165 \div 12 = 680 {5 \over 12} \]

Other Recommended Worksheets & Resources

Here are some of our other related worksheets you might want to look at.

Long Division Support

We have created a calculator to help you master the long division method.

Just type in the dividend and divisor and let the calculator show you how to work out the long division, step-by-step.

The calculator also gives commentary to show you what is happending at each stage.

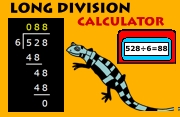
• Step by Step Long Division Calculator

More Randomly Generated Multiplication and Division Worksheets

As well as our long division problems with answers generator on this page, we also have a a generator for making your own division facts and several multiplication worksheet generators.

• Long Division with Money (randomly generated)
• Division Facts Worksheet Generator
• Single Digit Multiplication Worksheets Generator
• Multi Digit Multiplication Generator
• Money Multiplication Worksheets Generator

Graded (non-generated) Division Sheets

As well as our randomly generated long division worksheets, we also have a selection of carefully graded division worksheets for each grade.

These sheets are especially useful for introducing the concept and keeping the numbers to divide manageable and simple.

The sheets in the links below are not randomly generated, but have been carefully selected to progress your child through their division learning.

Learning long division starts at a 3rd grade level with learning to divide by a single digit. It then gradually gets more complex for higher grades.

Long Division Worksheets (graded)

• Long Division Worksheets 3rd Grade
• 4th Grade Long Division Worksheets
• Long Division Worksheets 5th Grade
• Long Division of Decimal Numbers

Mental Division Worksheets

As well as long division worksheets, you also need to know your division facts.

Knowing your division facts will help improve the speed and accuracy of your long division.

We have a wide variety of division fact worksheets for you to choose from.

Division Facts Worksheets

Division facts starts off with understanding the link between multiplication and division.

Once you understand this, you can start learning the division facts related to the multiplication table.

After this, at a 4th and 5th grade level, you can start learning related division facts.

This means that if you know that 12 ÷ 4 = 3 then you can work out 120 ÷ 4 = 30 or that 120 ÷ 40 = 3, etc

• Understanding Division Sheets (2nd grade)
• Division Facts to 10x10 Worksheets (3rd grade)
• Divding by Multiples of 10 and 100 Worksheets (4th grade)
• Decimal Division Facts (5th grade)

Multiply and Divide by 10 and 100

Knowing how to multiply and divide numbers by 10 and 100 is a very useful skill.

It is especially useful when converting between metric units or when using percentages.

• Multiply and Divide by 10 and 100 (decimals)

Division Math Games

Here you will find a range of Free Division Games.

The following games develop the Math skill of dividing and learning your division facts in a fun and motivating way.

Using these sheets will help your child to:

• learn their division facts;
• develop their strategic thinking skills.
• Math Division Games

Return to Division Worksheets

Return to Math Worksheets Generator

Return from Long Division Problems with Answers to Math Salamanders Home Page

Math-Salamanders.com

The Math Salamanders hope you enjoy using these free printable Math worksheets and all our other Math games and resources.

We welcome any comments about our site or worksheets on the Facebook comments box at the bottom of every page.

New! Comments

TOP OF PAGE

© 2010-2024 Math Salamanders Limited. All Rights Reserved.

• Privacy Policy
• Copyright Policy

KS2 Tutoring Programmes

Mastery-aligned lessons designed to plug individual gaps and delivered by specialist maths tutors

FREE daily maths challenges

A new KS2 maths challenge every day. Perfect as lesson starters - no prep required!

Long Division Questions That Will Test All Pupils From Year 6 To Year 11

Beki Christian

Long division questions are designed for students to practise dividing large numbers such as a four digit number by a two digit number or a three digit number. When larger numbers are involved, it is no longer practical to use short division (the bus stop method).

Here is a selection of long division questions you can share with your students to help them work through the steps involved in long division.

What is the long division method?

Long division question example, long division questions year 6, long division sats questions, long division questions ks3, long division questions ks4, looking for more long division questions and resources, long division key terminology.

Let’s recap some key points:

• The division symbol looks like this: ÷
• The dividend is the number we are dividing.
• The divisor is the number we are dividing by.
• The quotient is the quantity produced by the division.

We can think of long division as a step by step process in which the steps are repeated until the calculation is complete. It is best used when working with multi-digit divisors and dividends.

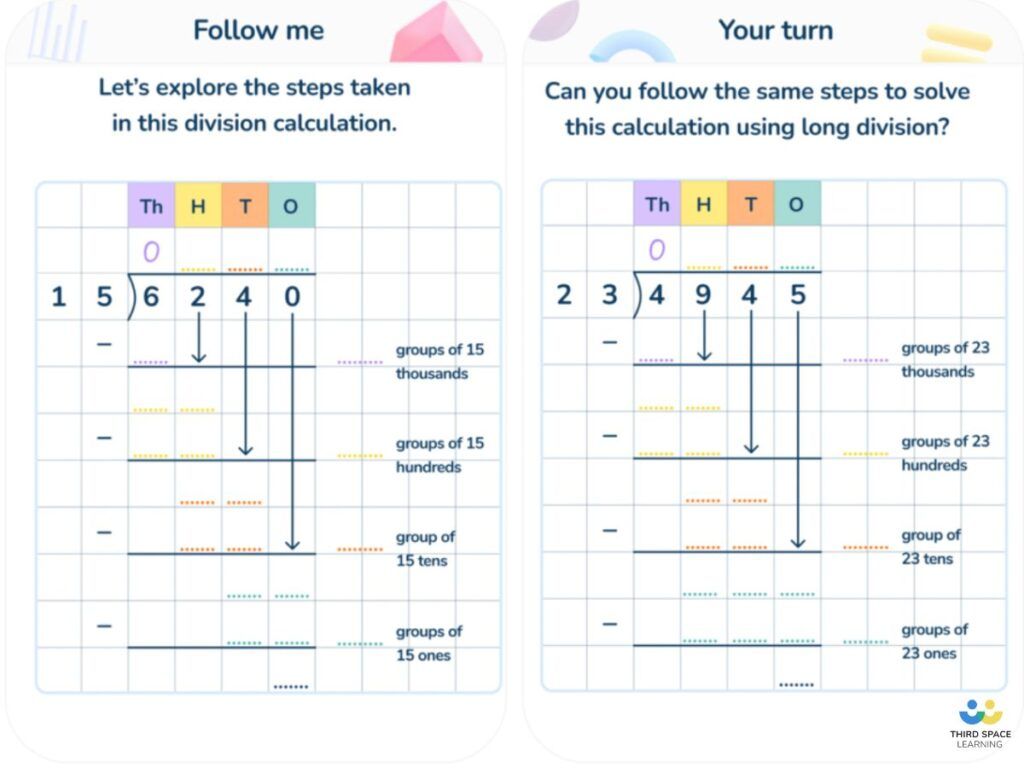
When completing a long division calculation, the first thing we do is write out the first 9 multiples of the divisor. This makes the next steps a lot easier. Once we have done this, we can follow these long division steps:

• Bring the next digit down

If you have been struggling to teach long division to your Year 6 class then this post on the long division method step by step will prove invaluable! 

3 Free Long Division Worksheets For KS2

Get some free, ready to use long division worksheets for your class, all of which were created by a primary teacher!

Let’s look at an example:

First write out the first 9 multiples of 24. This can be worked out either using partitioning or by repeated addition. By writing out the multiples of 24, you are saving yourself the time of working through the 24 times table mentally several times as well as ensuring you make less mistakes. 

Multiples of 24:

24, 48, 72, 96, 120, 144, 168, 192, 216, …

Now follow the steps:

• Divide : Divide 175 tens by 24. The answer is 7 – I can see this from my multiples, so I write 7 on my answer line in the tens column as the first digit of my answer.
• Multiply : 24 x 7 = 168 (Again I can see this from multiples). Ensure you align the place values correctly when placing this on the grid.
• Subtract : 175 – 168 = 7.
• Bring down : bring down the 2 from the ones column.
• Divide : Divide 72 by 24. The answer is 3 – I can see this from my multiples.
• Multiply : 24 x 3 = 72
• Subtract : 72 – 72 = 0
• The answer to the subtraction here is 0, therefore the remainder is zero and we are finished.

In some long division problems the divisor does not divide the dividend a whole number of times and in these cases the answer may be written with a remainder, a decimal or a fraction.

The national curriculum states that we need to be able to interpret remainders as whole number remainders, fractions, or by rounding, as appropriate for the context.

Long division questions KS2

Long division questions in upper KS2 typically involve 2 digit numbers as divisors. Students learn the process of long division and then apply the same method of how to divide numbers to reasoning and word problems. Before tackling long division problems, it is important that students have a good understanding of the basic multiplication and division facts.

1. Work out the answer to 595 ÷ 7

The multiples of 7 are:  7,  14,  21,  28,  35,  42,  49,  56,  63, …

2.  Calculate 4488 ÷ 34

The multiples of 34 are:  34,  68,  102,  136,  170,  204,  238,  272,  306,…

3. Work out 6720  ÷ 25

The multiples of 25 are:  25,  50,  75,  100,  125,  150,  175,  200,  225, …

4. Calculate 9129 ÷ 68 . Give your answer as a mixed number (a whole number and a fraction).

The multiples of 68 are:  68,  136,  204,  272,  340,  408,  476,  544,  612, …

5. What is the remainder when 3810 is divided by 47?

The multiples of 47 are:  47,  94,  141,  188,  235,  282,  329,  376,  423, …

6. Nadim is calculating 4482 ÷ 54. His calculation is shown below.

Nadim has made a mistake. Which of the circled numbers is incorrect?

0 is incorrect. He should have brought the 2 down.

7. A zoo charges £13 per ticket. In one day the zoo took £6,136 in ticket sales. How many people visited the zoo that day?

The calculation we need to do is £6,136 ÷ 13

The multiples of 13 are: 13, 26, 39, 52, 65, 78, 91, 104, 117, …

472 people visited the zoo that day.

8. Sophia is going to make some bracelets. Each bracelet uses 77 beads. Sophia has 3500 beads. How many bracelets can Sophia make?

We need to work out 3500 ÷ 77

The multiples of 77 are:  77,  154,  231,  308,  385,  462,  539,  616,  693, …

Sophia can make 45 bracelets. She will have 35 beads left over.

9. A shopkeeper sells pineapples for 93p each. One week he sells £91.14 worth of pineapples. How many pineapples did he sell that week?

£91.14 is the same as 9114p. In this case it makes more sense to multiply by 100 and work in pence than to work with decimals.

Multiples of 93:  93,  186,  279,  372,  465,  558,  651,  744,  837, …

He sold 98 pineapples.

10. Below is a calculation. Some numbers have been covered up.

What number should appear in place of the triangle?

Subtracting 136 from 1__0 gives us 54.

136 + 54 = 190, so the number under the square should be 9.

68 + 19 = 87, so the number under the triangle should be 7.

(The number under the circle should be 6 since 1 multiplied by the number gives us 68.)

For more KS2 division practice and additional long division questions, download these free practice worksheets .

Long division in KS3 and KS4

Long division is not taught explicitly in KS3 and KS4 but it can come in useful when solving division problems. It is expected that students can apply multiplication and division skills such as long multiplication and long division across all areas of the KS3 and KS4 curriculum. Below are some examples of questions from KS3 and KS4 where long division could be applied.

11. Lucy buys a car for £7500. She pays £4080 up front and splits the rest of the cost over 36 months. Work out how much she pays each month.

£7500 – £4080 = £3420.

She has £3420 to pay so we need to calculate £3420 ÷ 36

The multiples of 36 are:  36,  72,  108,  144,  180,  216,  252,  288,  324, …

She will pay £95 each month.

12.   The area of this rectangle is 1224cm ^2 .

Find the length of the base of the rectangle.

Since area = base × height , 1224 = base × 17

We need to calculate 1224 ÷ 17

The multiples of 17 are:  17,  34,  51,  68,  85,  102,  119,  136,  153,….

The length of the base is 72cm.

13. The probability of winning a game is \frac{3}{13} . If 845 people play the game, how many would you expect to win?

We need to calculate \frac{3}{13} of 845.

The multiples of 13 are:

13,  26,  39,  52,  65,  78,  91,  104,  117, …

65 × 3 = 195

You would expect 195 people to win.

14.  Solve the equation 23(2x + 1) = 1219

The multiples of 46 are:  46,  92,  138,  184,  230,  276,  322,  368,  414, …..

Third Space Learning’s free GCSE maths resource library contains detailed lessons with step-by-step instructions on how to solve long division problems at secondary, as well as long division worksheets with practice questions and more GCSE exam questions.

For primary school teachers, have a look at this guide on teaching division at KS2 and these long division examples to support your teaching.

DO YOU HAVE STUDENTS WHO NEED MORE SUPPORT IN MATHS?

Every week Third Space Learning’s maths specialist tutors support thousands of students across hundreds of schools with weekly online maths tuition designed to plug gaps and boost progress.

Since 2013 these personalised one to one lessons have helped over 169,000 primary and secondary students become more confident, able mathematicians.

Learn about the scaffolded lesson content or request a personalised quote for your school to speak to us about your school’s needs and how we can help.

Related articles

What Is Subitising And Why Is It Important? Tips For Parents & Teachers

What Is The Column Method Of Addition And Subtraction? Explained For Primary School

What Is BIDMAS: Explained for Primary School 

What Is Order Of Operations: Explained For Primary School 

FREE Long Division Worksheets for KS2

3 ready to use worksheets for your class that will help them with all aspects of long division from 1-digit numbers through to working out multiples!

One worksheet covering division with 1-digit numbers, one covering 2-digit numbers and one for working out multiples.

Privacy Overview

WELCOME!     Find what you need

Elementary Math

Elementary Ela-Reading

Teaching Tips

Career Exploration

How to solve long division problems.

Refresh your memory on how to teach long division with success for students and yourself. This step-by-step guide is filled with tips and helpful advice.

So many teachers become frustrated while teaching students how to solve long-division problems. I know that feeling well; I’ve been there. But teaching long division doesn’t have to be as difficult as that – cross my heart! 

I’ll walk you through a few steps that make long division easier for you to teach. But first, let’s talk to our students about what long division is and why it exists in the first place.

Introduce the topic to students.

As you’re introducing long division to students, setting the right tone is important. They shouldn’t be told how hard it is or that it’s scary and tough to learn. They need reassurance and confidence that they’ll be successful with your help.

Say something like, “I know you’re excited to learn about long division. We’ll use many skills you’ve learned over the years and put them together to solve division problems.”

Go on to explain that long division is simply a way of splitting a number into smaller, even parts. In real-life terms, it’s a way of dividing a big group of things into many smaller groups. 

For example, if we have a bag of candies and a group of children. We want each child to get the same number of candies, so we need to figure out how many candies each child gets. We can find the answer through long division.

Teach the basic terminology.

While instructing students, you should use the correct terminology. Here’s a quick refresher:

• The dividend is the number being divided or split up (the number inside the division symbol).
• The divisor is the number you divide by (the number of groups the dividend is split into).
• The quotient is the name of the answer to the division problem (how many are in each group).
• The remainder is the number that is left over after dividing.

In other words, dividend ÷ divisor = quotient , and the remainder is any extra amount.

You may have to provide several more examples to help students understand why we perform long division.

Long division can be broken down into steps.

Long division is a process that can be broken down into steps. Teaching each step will make it easier for students to become fluent. Long division takes practice, repetition, and feedback, but students will master the skill over time.

As you begin to instruct students on the steps for solving long division, follow these steps:

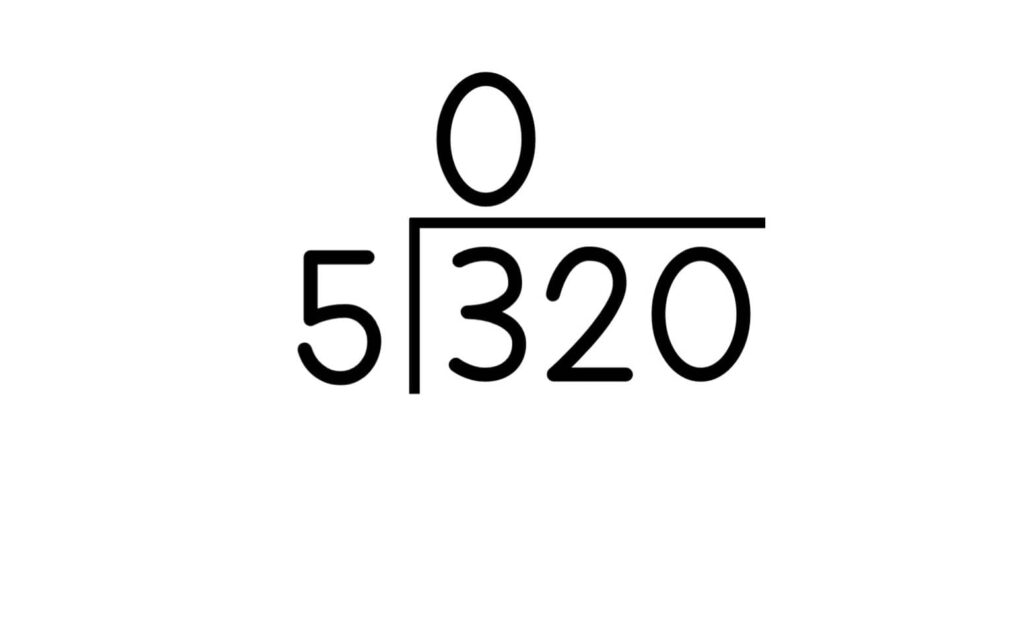
1. Set up the equation with a dividend and divisor. 

2. Divide the first digit in the dividend by the divisor by finding how many times the divisor can go into the first digit of the dividend. Write that number above the first digit of the dividend. That is the first digit of the quotient. In the above example, ask how many groups of 5 are there in 3?

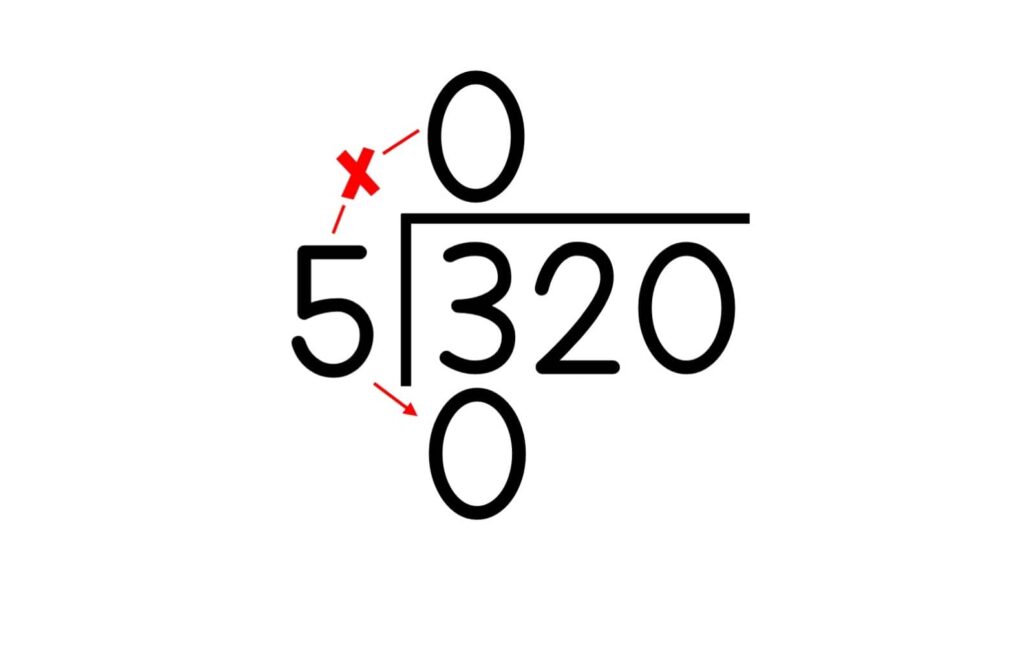
3. If the divisor is larger than the first digit, place a zero above the first digit of the dividend

4. Multiply the digit in the quotient times the divisor. In our example, this is 0.

5. Write the product below the dividend.

6. Write a line under the product.

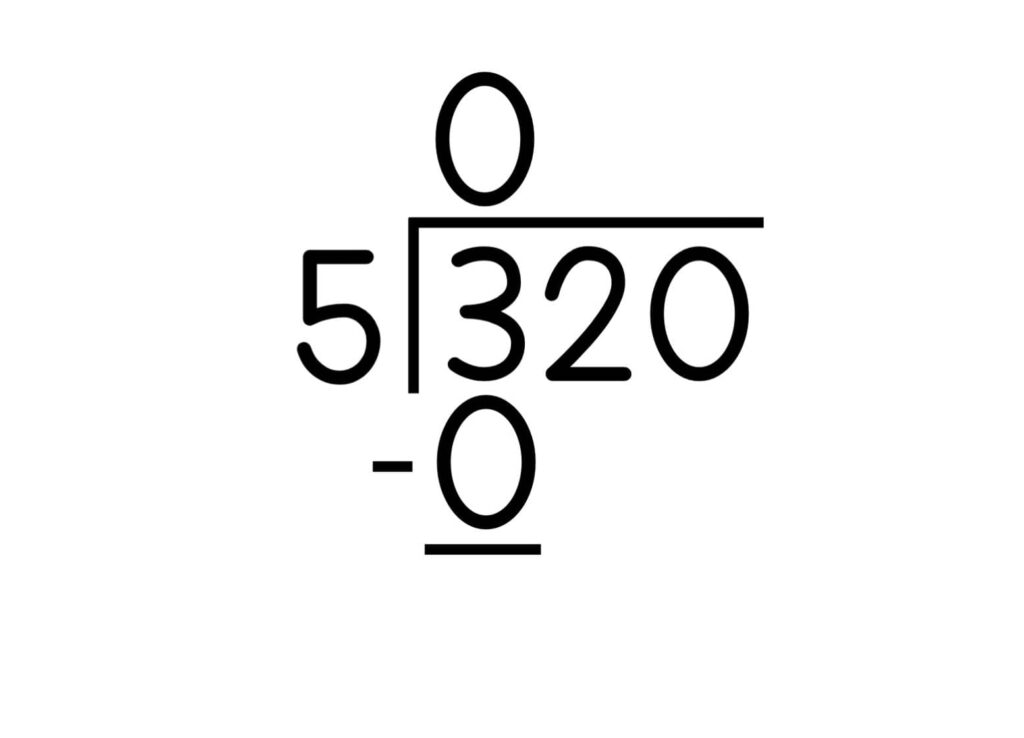
7. Subtract the product from the dividend. Write the difference (answer) under the line.

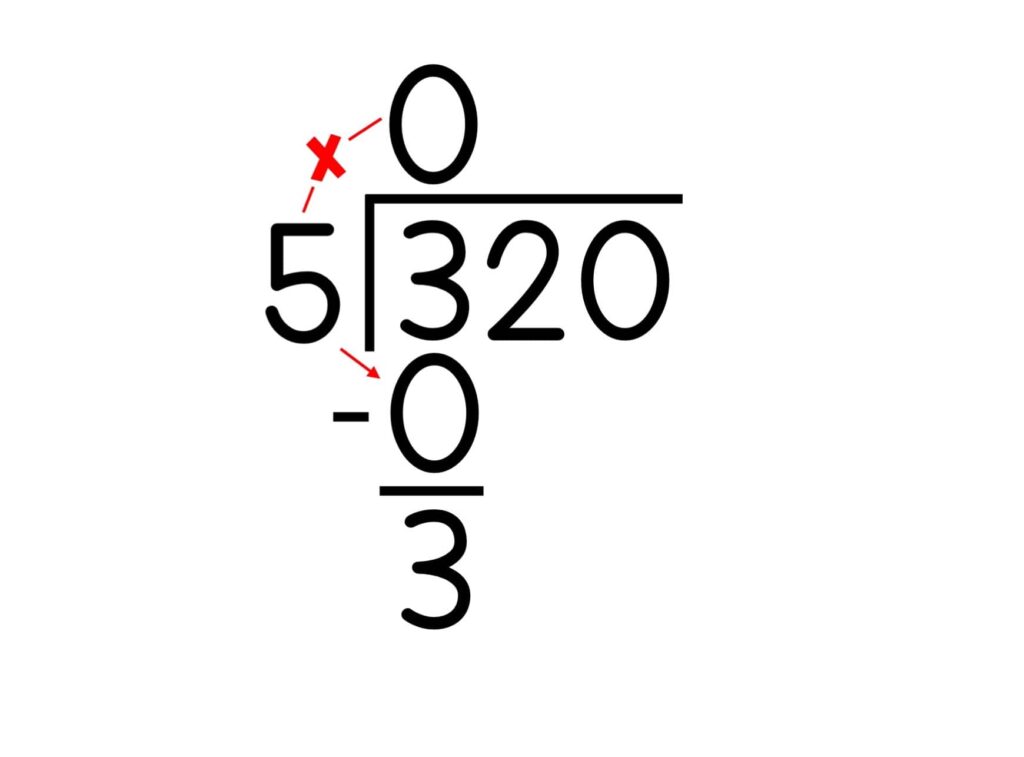
8. Bring down the next digit in the dividend.

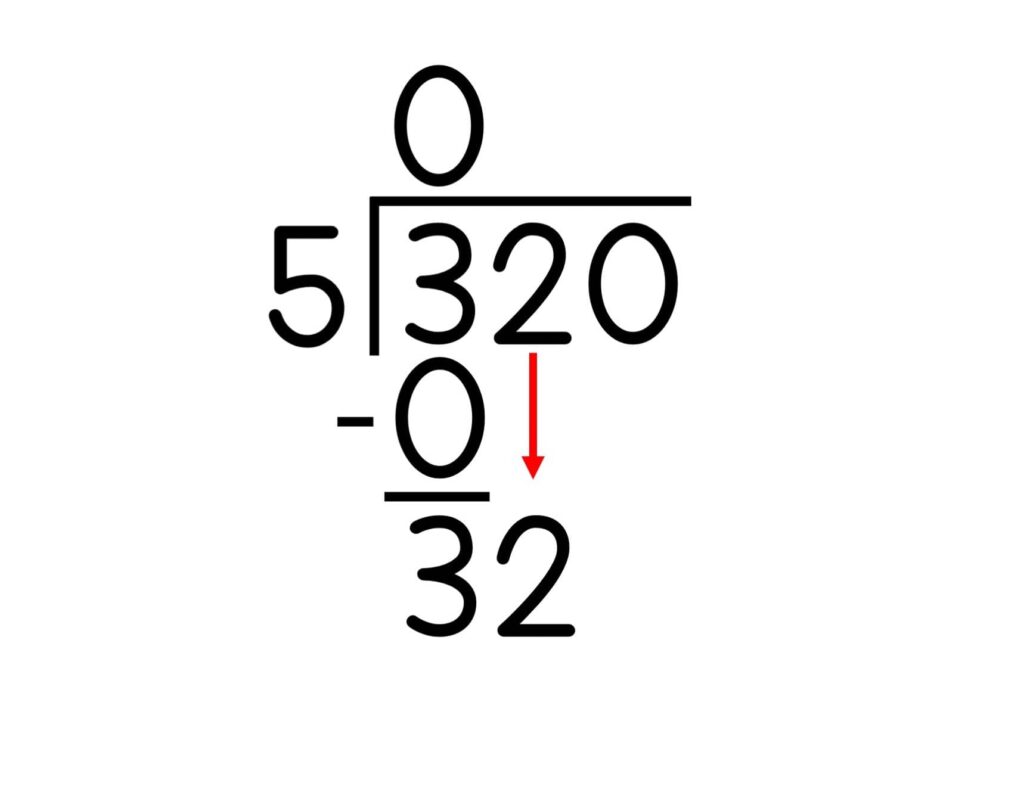
9. Repeat the process by dividing the new number by your divisor. Write that digit above the dividend as the next digit of the quotient. Continue this process until all the digits in the dividend have been brought down and divided.

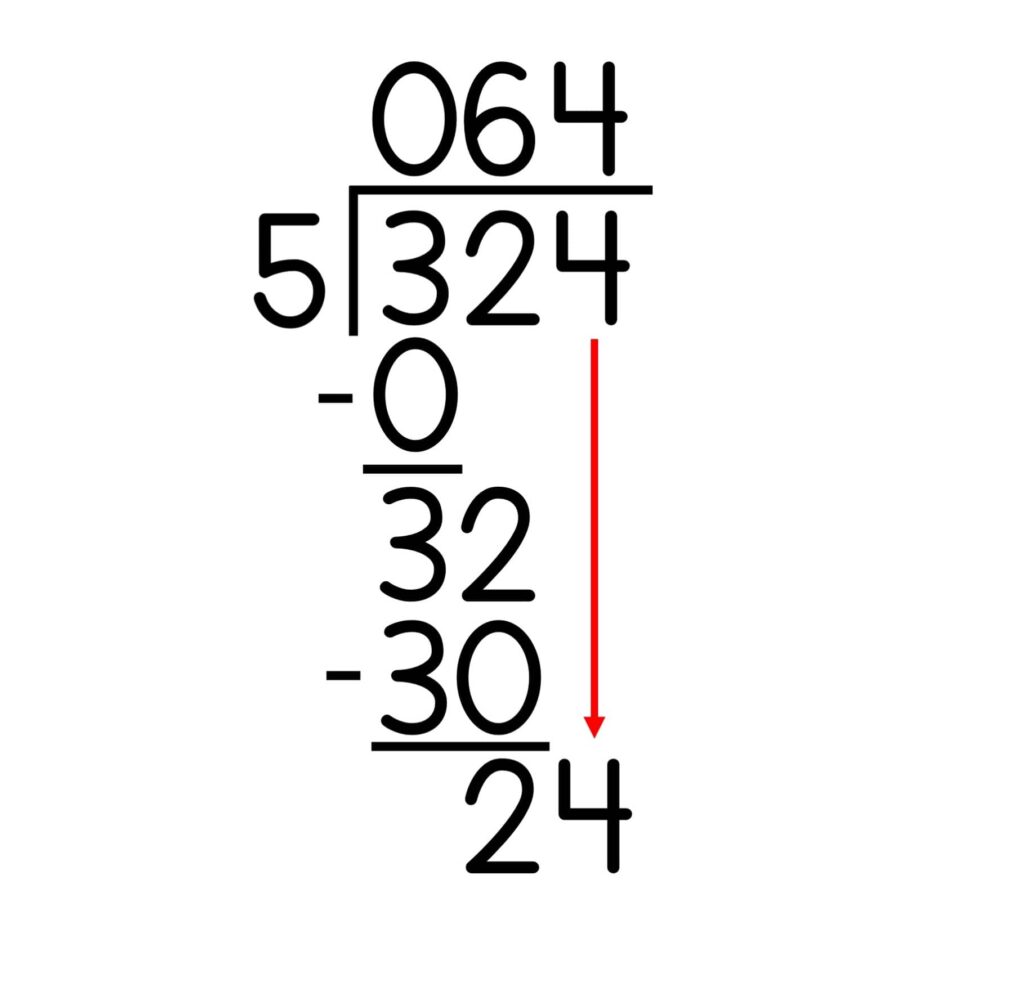
10. If the numbers don’t divide evenly, you’ll be left with a remainder. After the quotient, write “R.” and then the number left over. In our example, that remainder is 4.

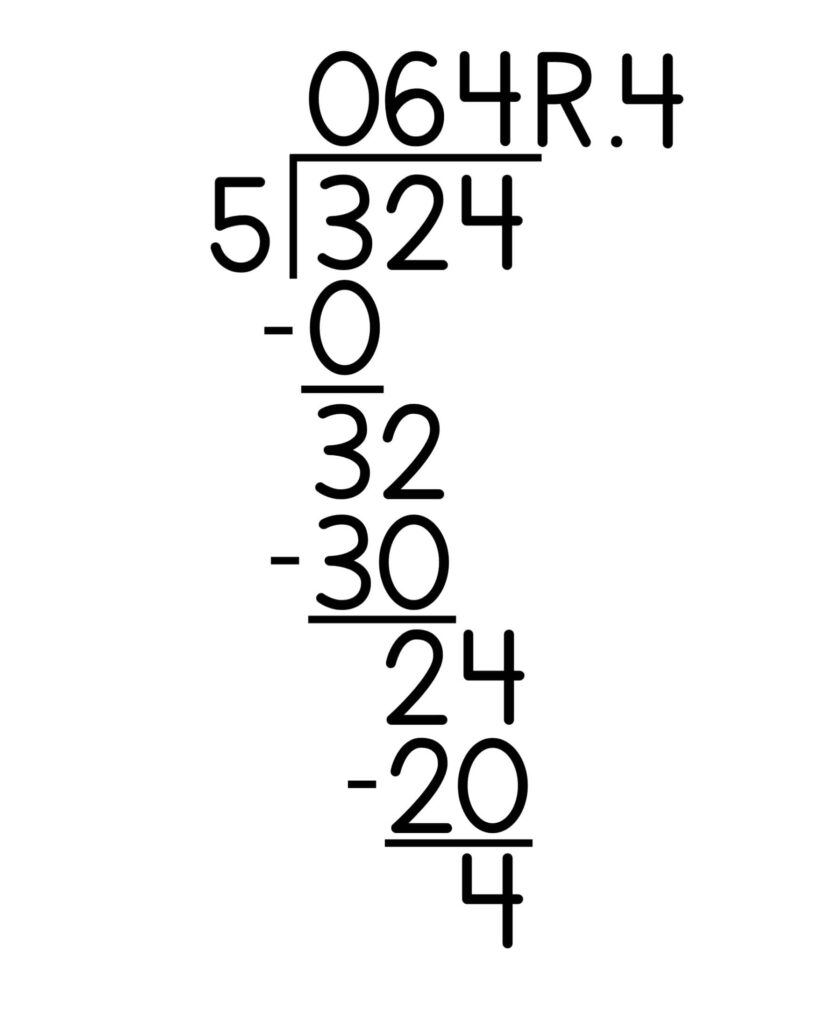
11. Remember to label your quotient and final answer. If you divide candies between children, you’ll label the final answer “candies.”

Provide several examples and think out loud.

Students learn a lot by hearing how you problem solve. 

As you’re explaining the examples, be sure to model your thinking out loud. For example, you might say, “Hmm. How many groups of 7 can be taken out of 30? I know 7 x 4 is 28, and that’s pretty close to 30.”

Be sure to:

• Over-teach by providing plenty of examples.
• Explain your thinking and reasoning for each step of the process.
• Be consistent. Repeat the process the same way every time.
• Provide real-world examples to help students understand how we use division in real-life situations.
• Use visual aids such as anchor charts, reference guides, posters, and diagrams to illustrate the concepts.
• Provide frequent feedback. The more often you provide feedback, the more students can self-correct.

Practice, practice, practice in a group.

Provide a long division problem for students to solve at their seats using whiteboards or paper. Have students practice problems independently or with assistance from a partner. As students are working, circulate around the room to provide frequent feedback. Once most students are finished, invite a student to show their work as they solve the problem in front of the class. Sometimes hearing the steps from a peer helps students understand. Discuss problem areas before moving on to the next problem.

As you’re circulating around the room, if you see students having trouble, ask them questions about their thinking processes. Make corrections on the spot to ensure that students understand the steps. 

You can also have students use their whiteboards for questions about what they don’t understand. 

More about partner work.

Partner work with whiteboards is a great way for students to learn from each other. They can also check each other’s work while practicing to improve their skills. Teaching others is the highest form of understanding, so students who are comfortable with the process can help classmates who are still struggling. Both students benefit. 

Important note – Peer tutoring can be a valuable part of learning for both students. However, don’t overuse it. Advanced students should be challenged so they continue moving forward. Struggling students need direct instruction from trained teachers. Use this approach wisely.

During partner work, you can circulate around the room and catch students’ mistakes immediately. The immediate feedback and reinforcement save students time and frustration. 

Be sure to note the students who need more assistance. You can work with those individuals in a small group or individually.

My favorite method for teaching long division.

My absolute favorite method for teaching long division is Shaped Math . It’s fantastic for introducing long division and for students who need support. 

Check out the video that explains this visual support for teaching long division.

Grab This FREE Long Division Set:

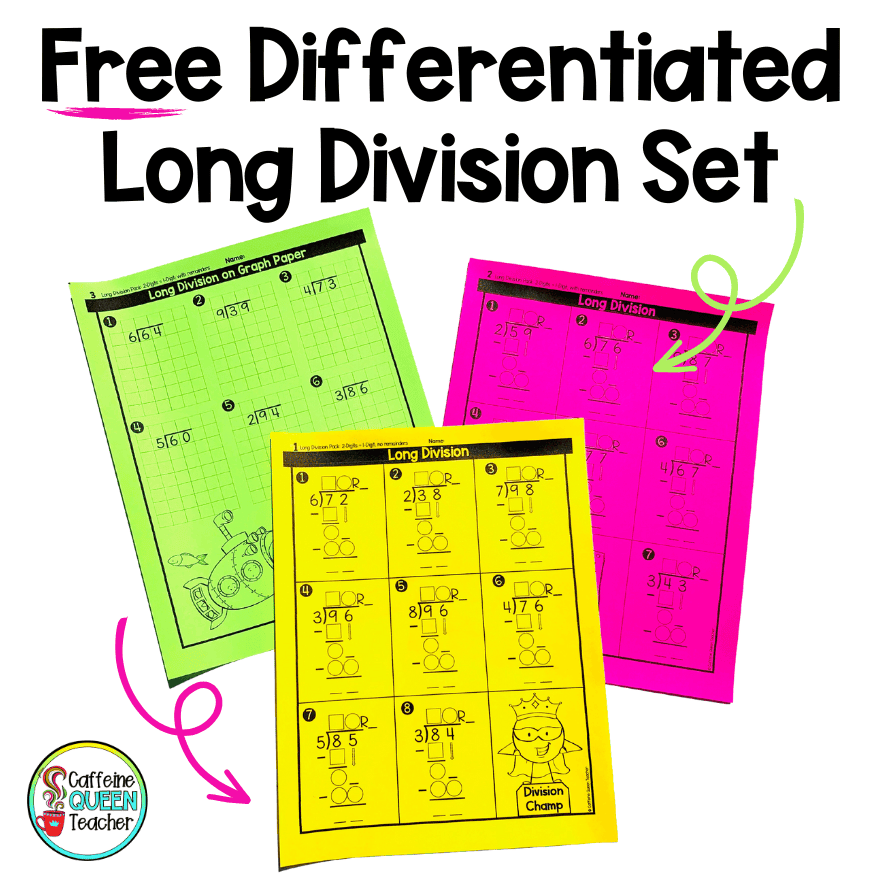
Grab the FREE Shaped Math Long Division.

Learn more about How to Teach Long Division with Shaped Math.

Additional tools to support students:

For students who need additional support, try out graph paper accommodations.

Standard Algorithm Using Shaped Math.

Conclusion .

Hopefully, you’ve found these tips helpful. If you’re teaching long division, this strategy is a great way for students to learn the skill successfully.

Hi, I’m Jules

Find it fast, browse the blog, visit my teachers pay teachers shop.

We're sorry, but we don't support Internet Explorer anymore. Please use a different browser .

Long Division

Online practice for grades 4-6.

Practice long division problems step-by-step interactively and online!

Each long division problem is presented in a grid, and you can write numbers and calculate the remainders just like if you were using paper and pencil.

You can choose the number of digits for the dividend and for the divisor (actually, a range for the number of digits), to make the problems easier or more challenging.

- For fourth grade: choose single-digit divisors and 2-4 digit dividends

- For fifth grade: choose single and two-digit divisors, and 3-6 digit dividends

At any point of your practice problem, you can push the "Check" button to check your work thus far. Any wrong digits are marked red, and correct ones are marked green.

Filter year:

Long Division 1 Reasoning and Problem Solving

Ensure a secure understanding of long division with this Year 6 Long Division 1 Reasoning and Problem Solving resource. It is differentiated into three levels of challenge to meet the needs of a range of learners and each worksheet includes six questions. Children will solve a word problem, identify the missing digits, and spot the errors in a calculation.

This resource could be used as a group task or independently. To take the stress and time out of marking, we've also provided the answers too.

Curriculum Objectives

• Divide numbers up to 4 digits by a two-digit number using the formal written method of short division where appropriate, interpreting remainders according to the context
• Solve problems involving addition, subtraction, multiplication and division

Calculations

More like this

More Y6 resources